Lamé parameters

In linear elasticity, the Lamé parameters are the two parameters

λ, also called Lamé's first parameter,
μ>0, the shear modulus or Lamé's second parameter, also denoted $G$ ,

which, in homogenous and isotropic materials, satisfy Hooke's law in 3D,

$\sigma=2\mu \varepsilon %2B\lambda \; \mathrm{tr}(\varepsilon)I$

where σ is the stress, ε the strain tensor, 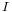$\scriptstyle I$ the identity matrix and 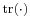$\scriptstyle\mathrm{tr}(\cdot)$ the trace function.

The first parameter λ is related to the bulk modulus and the shear modulus 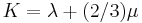$K = \lambda %2B (2/3) \mu$ , and serves to simplify the stiffness matrix in Hooke's law. Although the shear modulus, μ, must be positive, the Lamé's first parameter, λ, can be negative, in principle; however, for most materials it is also positive. The two parameters together constitute a parametrization of the elastic moduli for homogeneous isotropic media, popular in mathematical literature, and are thus related to the other elastic moduli.

The parameters are named after Gabriel Lamé.

References

K. Feng, Z.-C. Shi, Mathematical Theory of Elastic Structures, Springer New York, ISBN 0-387-51326-4, (1981)
G. Mavko, T. Mukerji, J. Dvorkin, The Rock Physics Handbook, Cambridge University Press (paperback), ISBN 0-521-54344-4, (2003)

Elastic moduli for homogeneous isotropic materials

Bulk modulus ( $K$ ) · Young's modulus ( $E$ ) · Lamé's first parameter ( $\lambda$ ) · Shear modulus ( $G$ ) · Poisson's ratio ( $\nu$ ) · P-wave modulus ( $M$ )

Conversion formulas
Homogeneous isotropic linear elastic materials have their elastic properties uniquely determined by any two moduli among these, thus given any two, any other of the elastic moduli can be calculated according to these formulas.
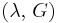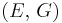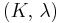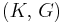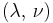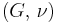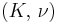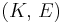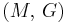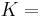	$(\lambda,\,G)$	$(E,\,G)$	$(K,\,\lambda)$	$(K,\,G)$	$(\lambda,\,\nu)$	$(G,\,\nu)$	$(E,\,\nu)$	$(K,\, \nu)$	$(K,\,E)$	$(M,\,G)$
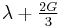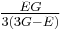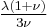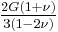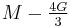$K=\,$	$\lambda%2B \tfrac{2G}{3}$	$\tfrac{EG}{3(3G-E)}$			$\tfrac{\lambda(1%2B\nu)}{3\nu}$	$\tfrac{2G(1%2B\nu)}{3(1-2\nu)}$	$\tfrac{E}{3(1-2\nu)}$			$M - \tfrac{4G}{3}$
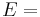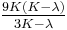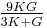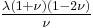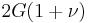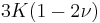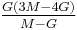$E=\,$	$\tfrac{G(3\lambda %2B 2G)}{\lambda %2B G}$		$\tfrac{9K(K-\lambda)}{3K-\lambda}$	$\tfrac{9KG}{3K%2BG}$	$\tfrac{\lambda(1%2B\nu)(1-2\nu)}{\nu}$	$2G(1%2B\nu)\,$		$3K(1-2\nu)\,$		$\tfrac{G(3M-4G)}{M-G}$
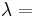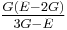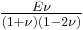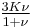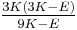$\lambda=\,$		$\tfrac{G(E-2G)}{3G-E}$		$K-\tfrac{2G}{3}$		$\tfrac{2 G \nu}{1-2\nu}$	$\tfrac{E\nu}{(1%2B\nu)(1-2\nu)}$	$\tfrac{3K\nu}{1%2B\nu}$	$\tfrac{3K(3K-E)}{9K-E}$	$M - 2G\,$
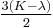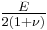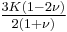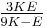$G=\,$			$\tfrac{3(K-\lambda)}{2}$		$\tfrac{\lambda(1-2\nu)}{2\nu}$		$\tfrac{E}{2(1%2B\nu)}$	$\tfrac{3K(1-2\nu)}{2(1%2B\nu)}$	$\tfrac{3KE}{9K-E}$
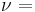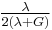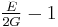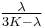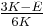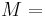$\nu=\,$	$\tfrac{\lambda}{2(\lambda %2B G)}$	$\tfrac{E}{2G}-1$	$\tfrac{\lambda}{3K-\lambda}$	$\tfrac{3K-2G}{2(3K%2BG)}$					$\tfrac{3K-E}{6K}$	$\tfrac{M - 2G}{2M - 2G}$
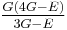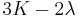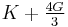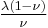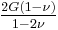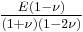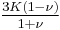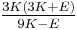$M=\,$	$\lambda%2B2G\,$	$\tfrac{G(4G-E)}{3G-E}$	$3K-2\lambda\,$	$K%2B\tfrac{4G}{3}$	$\tfrac{\lambda(1-\nu)}{\nu}$	$\tfrac{2G(1-\nu)}{1-2\nu}$	$\tfrac{E(1-\nu)}{(1%2B\nu)(1-2\nu)}$	$\tfrac{3K(1-\nu)}{1%2B\nu}$	$\tfrac{3K(3K%2BE)}{9K-E}$
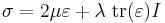
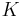